Highlight 2009-046Update time:12 01, 2009
Geophysical Prospecting, 2009, 57: 931-941 Lax-Wendroff and Nyström methods for seismic modelling Chen, Jing-Bo Abstract
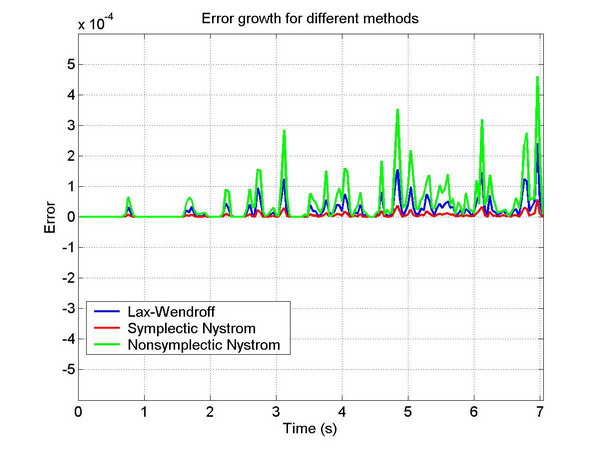
Lax-Wendroff and Nyström methods are numerical algorithms of temporal approximations for solving differential equations. These methods provide efficient algorithms for high-accuracy seismic modeling. In the context of spatial pseudospectral discretizations, I explore these two kinds of methods in a comparative way. Their stability and dispersion relation are discussed in detail. Comparison between the fourth-order Lax-Wendroff method and a fourth-order Nyström method shows that the Nyström method has smaller stability limit but has a better dispersion relation, which is closer to the sixth-order Lax-Wendroff method. The structure-preserving property of these methods is also revealed. The Lax-Wendroff methods are a second-order symplectic algorithm, which is independent of the order of the methods. This result is useful for understanding the error growth of Lax-Wendroff methods. Numerical experiments based on the scalar wave equation are performed to test the presented schemes and demonstrate the advantages of the symplectic methods over the nonsymplectic ones.
|
Contact
Related Articles
Reference
|
-
SIMSSecondary Ion Mass Spectrometer Laboratory
-
MC-ICPMSMultiple-collector ICPMS Laboratory
-
EM & TEMElectron Microprobe and Transmission Electron Microscope Laboratory
-
SISolid Isotope Laboratory
-
StIStable Isotope Laboratory
-
RMPARock-Mineral Preparation and Analysis
-
AAH40Ar/39Ar & (U-Th)/He Laboratory
-
EMLElectron Microscopy Laboratory
-
USCLUranium Series Chronology Laboratory
-
SASeismic Array Laboratory
-
SEELaboratory of Space Environment Exploration Laboratory
-
PGPaleomagnetism and Geochronology Laboratory
-
BioMNSFrance-China Bio-mineralization and Nano-structure Laboratory

 Print
Print Close
Close